Polar Rotation: Difference between revisions
No edit summary |
m (Reverted edits by Anti-Up (Talk); changed back to last version by Tolnaftate2004) |
||
| Line 1: | Line 1: | ||
''' | == Definitions == | ||
''θ'': greek letter ''theta''<br /> | |||
''φ'': greek letter ''phi''<br /> | |||
''theta'' and ''phi'' are commonly used in trigonometry for angles (but phi is most commonly used as a constant: 1.618...).<br /> | |||
''α'': 1<sup>st</sup> greek letter ''alpha''. For our purposes, it will represent rotation about the y-axis.<br /> | |||
''β'': 2<sup>nd</sup> greek letter ''beta''. For our purposes, it will represent rotation about the x-axis.<br /> | |||
''γ'': 3<sup>rd</sup> greek letter ''gamma''. For our purposes, it will represent rotation about the z-axis.<br /> | |||
''' | sin(''θ'') = y / ''r'' (Δy of a point from the center on a circle with radius ''r'') | ||
cos(''θ'') = x / ''r'' (Δx of a point from the center on a circle with radius ''r'') | |||
sin(''θ'' ± ''φ'') = sin(''θ'') cos(''φ'') ± cos(''θ'') sin(''φ'') | |||
-cos(''θ'' ± ''φ'') = -cos(''θ'') cos(''φ'') ± sin(''θ'') sin(''φ'') | |||
In addition (not really useful): | |||
tan(''θ'') = sin(''θ'') / cos(''θ'') | |||
cot(''θ'') = cos(''θ'') / sin(''θ'') | |||
sec(''θ'') = 1 / cos(''θ'') | |||
csc(''θ'') = 1 / sin(''θ'') | |||
== Deriving the 2D equations == | |||
Simply:<br /> | |||
x² + y² = ''r''²; the basic equation of a circle with center (0,0) | |||
x² + y² + z² = ''r''²; the basic equation of a sphere with center (0,0,0) | |||
sin²(''θ'') + cos²(''θ'') = 1 | |||
arctan(y/x) = ''θ''; arctan() being the inverse of tangent | |||
tan(arctan(y/x)) = tan(''θ'') | |||
y/x = tan(''θ'') | |||
y/x = ''r'' sin(''θ'') / ''r'' cos(''θ'') | |||
Thus,<br /> | |||
''r'' sin(''θ'') = y | |||
''r'' cos(''θ'') = x | |||
Likewise, | |||
sin(''θ'') = y / ''r'' | |||
cos(''θ'') = x / ''r'' | |||
Plugging into the circle equation, we get | |||
''r''² = ''r''² cos²(''θ'') + ''r''² sin²(''θ'') | |||
Compare: | |||
√(a² + b²) = c (the distance formula) | |||
x² + y² = ''r''² (established as the equation of a circle) | |||
√(a² + b²) = c | |||
( √(a² + b²) )² = c² | |||
a² + b² = c² | |||
== Rotation on a single plane == | |||
Think of a circle with some radius, ''r'', and a point somewhere on it. This circle is a two-dimensional figure on the xy [or y(x)] plane. The angle between 0º and the point is ''θ''. <br /> | |||
Trigonometric functions sine and cosine are specific to determine x and y values according the the angle passed as its parameter. <br /> | |||
To keep things simple, we will rotate about the (invisible) z-axis. In doing so, x and y are changing. Any z values that a point may have remain constant. ''φ'' is a second angle, representing the amount of rotation.<br /> | |||
y = ''r'' sin(''θ''), so, | |||
y = ''r'' sin(''θ'' + ''φ'') | |||
y = ''r'' ( sin(''θ'') cos(''φ'') + cos(''θ'') sin(''φ'') ) | |||
y = ''r'' sin(''θ'') cos(''φ'') + ''r'' cos(''θ'') sin(''φ'') | |||
Using substitutions form above, we can simplify to<br /> | |||
y = y cos(''φ'') + x sin(''φ'') | |||
Alternatively,<br /> | |||
x = ''r'' cos(''θ'') | |||
x = ''r'' cos(''θ'' + ''φ'') | |||
x = ''r'' ( cos(''θ'') cos(''φ'') - sin(''θ'') sin(''φ'') ) | |||
x = ''r'' cos(''θ'') cos(''φ'') - ''r'' sin(''θ'') sin(''φ'') | |||
x = x cos(''φ'') - y sin(''φ'') | |||
== Deriving the 3D equations == | |||
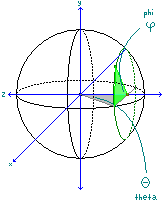
[[Image:Pointonsphere.png|thumb|right|This image shows how to determine the cartesian coordinates of a point on a sphere in terms of sine and cosine. The point is at the upper tip of the green triangle.]] | |||
As above, we can change the sphere's equation into a series of distance formulas | |||
x² + y² + z² = ''r''² | |||
( √(x² + y²) )² + z² = ''r''² | |||
( √( ( √(x² + y²) )² + z² ) )² = ''r''² | |||
√( x² + y² + z² ) = ''r'' | |||
''r''√( ( sin²(''θ'') + cos²(''θ'') ) sin²(''φ'') + cos²(''φ'') ) = ''r'' | |||
√( ( √( sin²(''θ'') + cos²(''θ'') ) )² sin²(''φ'') + cos²(''φ'') ) = 1 | |||
( sin²(''θ'') + cos²(''θ'') )² sin²(''φ'') + cos²(''φ'') = 1 | |||
sin²(''θ'') sin²(''φ'') + cos²(''θ'') sin²(''φ'') + cos²(''φ'') = 1 | |||
Comparing with the original equation we can split it up and get individual equations for x,y, and z. | |||
y = sin(''θ'') sin(''φ'') <sup>(1)</sup> | |||
x = cos(''θ'') sin(''φ'') <sup>(1)</sup> | |||
z = cos(''φ'') | |||
The equation is | |||
( sin²(''θ'') + cos²(''θ'') ) ( sin²(''φ'') + cos²(''φ'') ) = 1 | |||
So, why doesn't the ( sin²(''θ'') + cos²(''θ'') ) distribute to the cos²(''φ'')? If you'll recall, ( sin²(''θ'') + cos²(''θ'') ) = 1, so it ''could be'' that it was. But also, it was left out so that there were only 3 terms as opposed to 4 (We only want three: x, y, and z!). | |||
<hr width="33%" NOSHADE> | |||
<sup>(1)</sup> The portion used contained the original x or y equation, so was split up to match, but it doesn't matter in the end. | |||
== Rotation on three planes == | |||
Now in three dimensions:<br /> | |||
Imagine three planes that intersect: xy [y(x)], yz [z(y)], zx [x(z)] (using these names helps remember the order of calculations if re-writing the script... you'll see). <br /> | |||
For this, instead of merely one rotation angle, we will need three (''α'', ''β'', and ''γ''). Also, we will need to replace the simple x and y with their three-dimensional counterparts and add a z (To begin, ''f'' = ''f''<sub>0</sub>, where ''f'' is a function, x, y, or z).<br /> | |||
x<sub>0</sub> = ''r'' sin(''α'') cos(''β'') | |||
y<sub>0</sub> = ''r'' sin(''α'') sin(''β'') | |||
z<sub>0</sub> = ''r'' cos(''α'') | |||
About the x-axis:<br /> | |||
Finally, rotate our three planes to replace z with x, y with z, and x with y.<br /> | |||
nz = z cos(''β'') + y sin(''β'') | |||
ny = y cos(''β'') - z sin(''β'') | |||
Note how as the axes are switched, the x, y, and z or replaced respectively from the 2D rotation equations! | |||
We then replace y with ny and z with nz. So in a sense,<br /> | |||
z = nz | |||
y = ny | |||
About the y-axis:<br /> | |||
Now imagine the 3 planes rotate forward 90º and 90º to the left. The z has taken the place of the x, x in the stead of y, and y in that of z. The idea of this rotation is now just like that above, but y is constant.<br /> | |||
nx = x cos(''α'') + z sin(''α'') | |||
nz = z cos(''α'') - x sin(''α'') | |||
x = nx | |||
The two-dimensions calculations work for rotations about the z-axis.<br /> | |||
About the z-axis:<br /> | |||
ny = y cos(''γ'') + x sin(''γ'') | |||
nx = x cos(''γ'') - y sin(''γ'') | |||
We expand to simplify:<br /> | |||
x = x cos(''γ'') - y sin(''γ'') | |||
x = ( x<sub>0</sub> cos(''α'') + ( z<sub>0</sub> cos(''β'') + y<sub>0</sub> sin(''β'') ) sin(''α'') ) cos(''γ'') - ( y<sub>0</sub> cos(''β'') - z<sub>0</sub> sin(''β'') ) sin(''γ'') | |||
x = x<sub>0</sub> cos(''α'') cos(''γ'') + z<sub>0</sub> cos(''β'') sin(''α'') cos(''γ'') + y<sub>0</sub> sin(''β'') sin(''α'') cos(''γ'') - y<sub>0</sub> cos(''β'') sin(''γ'') + z<sub>0</sub> sin(''β'') sin(''γ'') | |||
x = x<sub>0</sub> cos(''α'') cos(''γ'') + y<sub>0</sub> ( sin(''β'') sin(''α'') cos(''γ'') - cos(''β'') sin(''γ'') ) + z<sub>0</sub> ( cos(''β'') sin(''α'') cos(''γ'') + sin(''β'') sin(''γ'') ) | |||
y = y cos(''γ'') + x sin(''γ'') | |||
y = ( y<sub>0</sub> cos(''β'') - z<sub>0</sub> sin(''β'') ) cos(''γ'') + ( x<sub>0</sub> cos(''α'') + ( z<sub>0</sub> cos(''β'') + y<sub>0</sub> sin(''β'') ) sin(''α'') ) sin(''γ'') | |||
y = y<sub>0</sub> cos(''β'') cos(''γ'') - z<sub>0</sub> sin(''β'') cos(''γ'') + x<sub>0</sub> cos(''α'') sin(''γ'') + z<sub>0</sub> cos(''β'') sin(''α'') sin(''γ'') + y<sub>0</sub> sin(''β'') sin(''α'') sin(''γ'') | |||
y = x<sub>0</sub> cos(''α'') sin(''γ'') + y<sub>0</sub> ( cos(''β'') cos(''γ'') + sin(''β'') sin(''α'') sin(''γ'') ) + z<sub>0</sub> ( -sin(''β'') cos(''γ'') + cos(''β'') sin(''α'') sin(''γ'') ) | |||
z = z cos(''α'') - x sin(''α'') | |||
z = ( z<sub>0</sub> cos(''β'') + y<sub>0</sub> sin(''β'') ) cos(''α'') - x<sub>0</sub> sin(''α'') | |||
z = z<sub>0</sub> cos(''β'') cos(''α'') + y<sub>0</sub> sin(''β'') cos(''α'') - x<sub>0</sub> sin(''α'') | |||
As is, the object will display in an orthographic projection, which means that z has no bearing on the perspective. To give a perspective projection, the x and y can be distorted by some equation like:<br /> | |||
x = centerx + h / (h-z) | |||
y = centery - h / (h-z) | |||
== Links == | |||
[http://forums.graalonline.com/forums/showthread.php?t=54167 Amon-ra's explanation and models (continues on p. 2)] | |||
Revision as of 08:19, 1 July 2007
Definitions
θ: greek letter theta
φ: greek letter phi
theta and phi are commonly used in trigonometry for angles (but phi is most commonly used as a constant: 1.618...).
α: 1st greek letter alpha. For our purposes, it will represent rotation about the y-axis.
β: 2nd greek letter beta. For our purposes, it will represent rotation about the x-axis.
γ: 3rd greek letter gamma. For our purposes, it will represent rotation about the z-axis.
sin(θ) = y / r (Δy of a point from the center on a circle with radius r) cos(θ) = x / r (Δx of a point from the center on a circle with radius r) sin(θ ± φ) = sin(θ) cos(φ) ± cos(θ) sin(φ) -cos(θ ± φ) = -cos(θ) cos(φ) ± sin(θ) sin(φ)
In addition (not really useful):
tan(θ) = sin(θ) / cos(θ) cot(θ) = cos(θ) / sin(θ) sec(θ) = 1 / cos(θ) csc(θ) = 1 / sin(θ)
Deriving the 2D equations
Simply:
x² + y² = r²; the basic equation of a circle with center (0,0) x² + y² + z² = r²; the basic equation of a sphere with center (0,0,0) sin²(θ) + cos²(θ) = 1
arctan(y/x) = θ; arctan() being the inverse of tangent tan(arctan(y/x)) = tan(θ) y/x = tan(θ) y/x = r sin(θ) / r cos(θ)
Thus,
r sin(θ) = y r cos(θ) = x
Likewise,
sin(θ) = y / r cos(θ) = x / r
Plugging into the circle equation, we get
r² = r² cos²(θ) + r² sin²(θ)
Compare:
√(a² + b²) = c (the distance formula) x² + y² = r² (established as the equation of a circle)
√(a² + b²) = c ( √(a² + b²) )² = c² a² + b² = c²
Rotation on a single plane
Think of a circle with some radius, r, and a point somewhere on it. This circle is a two-dimensional figure on the xy [or y(x)] plane. The angle between 0º and the point is θ.
Trigonometric functions sine and cosine are specific to determine x and y values according the the angle passed as its parameter.
To keep things simple, we will rotate about the (invisible) z-axis. In doing so, x and y are changing. Any z values that a point may have remain constant. φ is a second angle, representing the amount of rotation.
y = r sin(θ), so, y = r sin(θ + φ) y = r ( sin(θ) cos(φ) + cos(θ) sin(φ) ) y = r sin(θ) cos(φ) + r cos(θ) sin(φ)
Using substitutions form above, we can simplify to
y = y cos(φ) + x sin(φ)
Alternatively,
x = r cos(θ) x = r cos(θ + φ) x = r ( cos(θ) cos(φ) - sin(θ) sin(φ) ) x = r cos(θ) cos(φ) - r sin(θ) sin(φ) x = x cos(φ) - y sin(φ)
Deriving the 3D equations
As above, we can change the sphere's equation into a series of distance formulas
x² + y² + z² = r² ( √(x² + y²) )² + z² = r² ( √( ( √(x² + y²) )² + z² ) )² = r² √( x² + y² + z² ) = r r√( ( sin²(θ) + cos²(θ) ) sin²(φ) + cos²(φ) ) = r √( ( √( sin²(θ) + cos²(θ) ) )² sin²(φ) + cos²(φ) ) = 1 ( sin²(θ) + cos²(θ) )² sin²(φ) + cos²(φ) = 1 sin²(θ) sin²(φ) + cos²(θ) sin²(φ) + cos²(φ) = 1
Comparing with the original equation we can split it up and get individual equations for x,y, and z.
y = sin(θ) sin(φ) (1) x = cos(θ) sin(φ) (1) z = cos(φ)
The equation is
( sin²(θ) + cos²(θ) ) ( sin²(φ) + cos²(φ) ) = 1
So, why doesn't the ( sin²(θ) + cos²(θ) ) distribute to the cos²(φ)? If you'll recall, ( sin²(θ) + cos²(θ) ) = 1, so it could be that it was. But also, it was left out so that there were only 3 terms as opposed to 4 (We only want three: x, y, and z!).
(1) The portion used contained the original x or y equation, so was split up to match, but it doesn't matter in the end.
Rotation on three planes
Now in three dimensions:
Imagine three planes that intersect: xy [y(x)], yz [z(y)], zx [x(z)] (using these names helps remember the order of calculations if re-writing the script... you'll see).
For this, instead of merely one rotation angle, we will need three (α, β, and γ). Also, we will need to replace the simple x and y with their three-dimensional counterparts and add a z (To begin, f = f0, where f is a function, x, y, or z).
x0 = r sin(α) cos(β) y0 = r sin(α) sin(β) z0 = r cos(α)
About the x-axis:
Finally, rotate our three planes to replace z with x, y with z, and x with y.
nz = z cos(β) + y sin(β) ny = y cos(β) - z sin(β)
Note how as the axes are switched, the x, y, and z or replaced respectively from the 2D rotation equations!
We then replace y with ny and z with nz. So in a sense,
z = nz y = ny
About the y-axis:
Now imagine the 3 planes rotate forward 90º and 90º to the left. The z has taken the place of the x, x in the stead of y, and y in that of z. The idea of this rotation is now just like that above, but y is constant.
nx = x cos(α) + z sin(α) nz = z cos(α) - x sin(α)
x = nx
The two-dimensions calculations work for rotations about the z-axis.
About the z-axis:
ny = y cos(γ) + x sin(γ) nx = x cos(γ) - y sin(γ)
We expand to simplify:
x = x cos(γ) - y sin(γ) x = ( x0 cos(α) + ( z0 cos(β) + y0 sin(β) ) sin(α) ) cos(γ) - ( y0 cos(β) - z0 sin(β) ) sin(γ) x = x0 cos(α) cos(γ) + z0 cos(β) sin(α) cos(γ) + y0 sin(β) sin(α) cos(γ) - y0 cos(β) sin(γ) + z0 sin(β) sin(γ) x = x0 cos(α) cos(γ) + y0 ( sin(β) sin(α) cos(γ) - cos(β) sin(γ) ) + z0 ( cos(β) sin(α) cos(γ) + sin(β) sin(γ) )
y = y cos(γ) + x sin(γ) y = ( y0 cos(β) - z0 sin(β) ) cos(γ) + ( x0 cos(α) + ( z0 cos(β) + y0 sin(β) ) sin(α) ) sin(γ) y = y0 cos(β) cos(γ) - z0 sin(β) cos(γ) + x0 cos(α) sin(γ) + z0 cos(β) sin(α) sin(γ) + y0 sin(β) sin(α) sin(γ) y = x0 cos(α) sin(γ) + y0 ( cos(β) cos(γ) + sin(β) sin(α) sin(γ) ) + z0 ( -sin(β) cos(γ) + cos(β) sin(α) sin(γ) )
z = z cos(α) - x sin(α) z = ( z0 cos(β) + y0 sin(β) ) cos(α) - x0 sin(α) z = z0 cos(β) cos(α) + y0 sin(β) cos(α) - x0 sin(α)
As is, the object will display in an orthographic projection, which means that z has no bearing on the perspective. To give a perspective projection, the x and y can be distorted by some equation like:
x = centerx + h / (h-z) y = centery - h / (h-z)